Unveiling the Foundations: Examples of Undefined Geometry Terms
Imagine trying to build a house without a foundation. Impossible, right? In the realm of geometry, undefined terms serve as that crucial foundation. These terms, while lacking strict formal definitions, are the building blocks upon which all other geometric concepts are built. Their significance lies not in precise wording, but in the intuitive understanding they provide, allowing us to visualize and describe the world around us in terms of shapes, sizes, and positions.
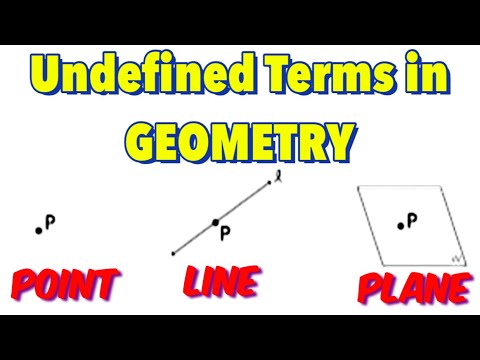
The most fundamental of these undefined terms are points, lines, and planes. We encounter them constantly in our everyday lives, even if we don't explicitly think of them in geometric terms. A tiny speck of dust in the air can represent a point, a seemingly endless stretch of highway embodies the concept of a line, and the smooth surface of a tabletop gives us a tangible idea of a plane.
The history of these undefined terms can be traced back to ancient civilizations. The Egyptians, for instance, used their understanding of lines and planes to construct their magnificent pyramids with astonishing precision. The Greeks, particularly Euclid, formalized the study of geometry with his groundbreaking work, "Elements." In it, he presented a system of axioms and postulates, many of which implicitly relied on these undefined terms.
While we may not be able to define these terms in the strictest sense, we can certainly describe them. A point can be thought of as a specific location in space, with no size or dimension. A line, on the other hand, extends infinitely in both directions and is often visualized as a straight path. Finally, a plane is a flat, two-dimensional surface that extends infinitely in all directions.
The importance of these undefined terms lies in their ability to provide a common starting point for understanding more complex geometric concepts. From defining angles and shapes to exploring the properties of triangles and circles, everything in geometry ultimately stems from these fundamental building blocks. Without them, the intricate world of geometric reasoning would simply crumble.
Let's consider some other key undefined terms in geometry:
- Space: The set of all possible points. It's the boundless "container" in which all geometric figures exist.
- Betweenness: This term describes the relative position of points on a line. We intuitively understand that point B lies between points A and C on a line segment AC.
These undefined terms, while seemingly simple, form the bedrock upon which the entire edifice of geometric knowledge is built. They demonstrate the power of intuitive understanding in mathematics, reminding us that sometimes, the most fundamental concepts are best left undefined, free to be interpreted and applied in countless ways.
Advantages and Disadvantages of Relying on Undefined Terms in Geometry
| Advantages | Disadvantages |
|---|---|
| Provides a common foundation for building geometric concepts. | Can lead to ambiguity if not handled carefully. |
| Allows for intuitive understanding and visualization of geometric ideas. | Makes it difficult to formally prove certain geometric theorems. |
| Simplifies the study of geometry by avoiding circular definitions. | Requires careful and consistent use of these terms to avoid contradictions. |
Common Questions About Undefined Terms in Geometry
Here are some common questions and answers that often arise when discussing undefined terms in geometry:
Q: If we can't define these terms, how can we use them in geometry?
A: While we don't have formal definitions, we have clear descriptions and intuitive understandings of what these terms represent. These descriptions are sufficient to use them as building blocks for other definitions and theorems.
Q: Are there other examples of undefined terms in mathematics?
A: Yes, set theory, another fundamental area of mathematics, also relies on undefined terms like "set" and "element."
Understanding the role of undefined terms in geometry is crucial for appreciating the elegance and logical structure of this fascinating field. By embracing these fundamental concepts, we open the door to a world of shapes, patterns, and spatial reasoning, enriching our understanding of the universe we inhabit.
My hero academia season 6s second ending a turning point for heroes
Unlocking math exploring saxon math 76
Unveiling the darkness a look at fate stay night heavens feel sub indo
