Unlocking Real-World Problems with Right Triangle Trigonometry
Have you ever looked at a tall building and wondered how tall it actually is? Or maybe you've seen a ramp and been curious about its angle? These real-world questions and countless others can be answered using the principles of right triangle trigonometry. It's a branch of mathematics that goes beyond textbook formulas and empowers us to understand and measure the world around us.
Right triangle trigonometry centers around the relationships between the angles and sides of a right triangle. We use trigonometric ratios – sine, cosine, and tangent, often shortened to sin, cos, and tan – to establish these relationships. These ratios allow us to calculate unknown side lengths or angles within a right triangle, given sufficient information.
The roots of trigonometry can be traced back to ancient civilizations like the Egyptians and Babylonians, who used its principles for surveying land and constructing pyramids. The development of trigonometry as a formal branch of mathematics is attributed to ancient Greek mathematicians. Over centuries, it has evolved into an essential tool in various fields, including engineering, architecture, physics, navigation, and even video game design.
The true power of right triangle trigonometry lies in its ability to solve real-world problems. Take, for instance, a surveyor needing to measure the width of a river. By setting up a right triangle with a known distance on one side and measuring an angle, they can use trigonometry to calculate the river's width without physically crossing it.
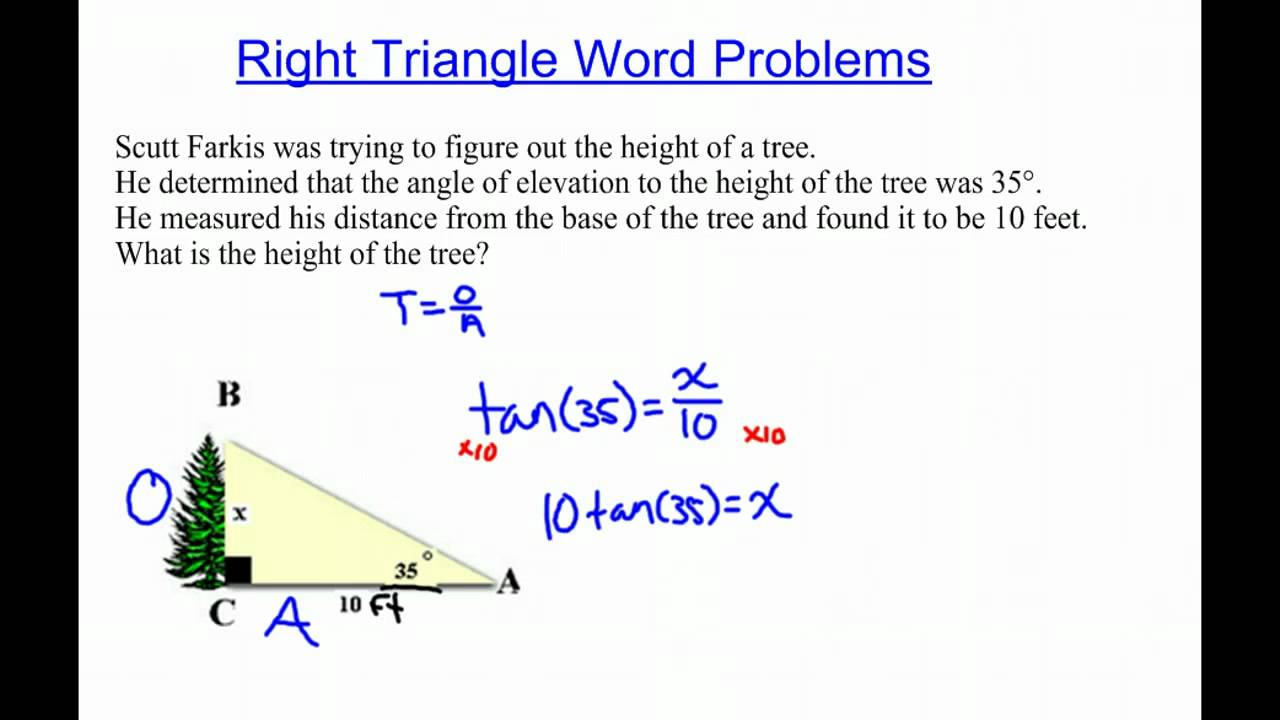
Let's illustrate this with a simple example. Imagine you're standing 50 feet away from a tree, and you want to determine its height. You measure the angle of elevation from your eye level to the top of the tree and find it to be 30 degrees. Using the tangent function (tan 30° = opposite side / adjacent side), you can calculate the height of the tree.
Advantages and Disadvantages of Learning Right Triangle Trigonometry
While tremendously useful, learning and applying right triangle trigonometry can have its advantages and disadvantages:
| Advantages | Disadvantages |
|---|---|
| Provides practical problem-solving skills applicable to numerous fields. | Can be challenging to grasp initially, especially for those unfamiliar with mathematical concepts. |
| Helps develop critical thinking and spatial reasoning abilities. | Requires memorization of formulas and understanding of trigonometric ratios. |
Despite the potential challenges, the ability to solve real-world problems using right triangle trigonometry makes it a rewarding and valuable skill to acquire.
To delve deeper into this topic, you can find a wealth of resources online, including practice problems and video tutorials. Websites like Khan Academy offer comprehensive lessons and practice exercises. Additionally, many textbooks dedicated to trigonometry can provide in-depth explanations and numerous examples to enhance your understanding.
Whether you're a student learning trigonometry for the first time or a professional seeking to refresh your knowledge, mastering the concepts of right triangle trigonometry opens a world of possibilities. Its applications are vast and varied, making it a truly fascinating and essential area of mathematics.
Unlocking the world of music your guide to free sheet music
Unlock your potential template resume spm malaysia guide
Closest tube station to madame tussauds